
|
E C G Sudarshan was born in 1931 in Kottayam, in Kerala and he took his B.Sc. (Honours) in Physics from Madras Christian College, Chennai, in 1951. He took his M.Sc. the following year and shifted to the Tata Institute of Fundamental Research, Mumbai. At TIFR, he worked with leading men, including Homi Bhabha. He worked on cosmic rays, an area where high energy radiation from outer space was revealing astonishing new results. During the years at TIFR Sudarshan also came into contact with George Marshak, with whom he was to do memorable work a few years later. |
|---|
|
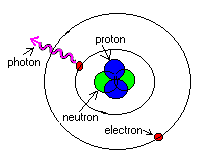
The 20th century had set out with just three particles as components of the atom, the positively charged proton, the negatively charged electron and the neutron, a neutral particle. Another particle known was the photon, the particle of light. The nucleus of the atom was known to consist of protons and neutrons, bound together by a short range, attractive force. Around the nucleus, which, which was positive , because of the protons, there orbited the electrons, in ‘shells’, that had successively increasing levels of energy. When the electrons transited from one energy level to another, they absorbed or emitted photons of the correct energy that separated the levels. |
|
|---|
Just these four ‘elementary’ particles participated in all radioactive decay of nuclei. Three kinds of decay were known. ‘Alpha’ decay was when groups of two protons and two neutrons, which is the constitution of the nucleus of the helium atom, escaped from a heavy nucleus, which typically consisted of hundreds of particles. Another form of radioactivity was the emission of high-energy photons, the gamma rays, when the nucleus went from a higher energy level to a lower level. And the third form of decay, known as beta decay, was when a neutron got converted into a proton, with the emission of an electron, to conserve the charge.
Alpha decay was not very complicated to explain, in terms of economics of binding energy of nuclei. The protons in the nucleus are positively charged, and they repel each other. As the particles have been brought together, against repulsion, this would have cost some energy. When squeezed lose enough together, an attractive ‘strong’ force becomes active and then keeps them together, something like a golf hole at the top of a hill keeps the golf ball safely inside once the ball has been sent all the way up the hill, to fall in.
In quantum mechanics, there is always a probability for the ‘golf ball’ to pop out of the hole and then roll down the hill, with a ‘temporary violation’ of the common laws of physics. In the case of a large nucleus, some of the nucleons are pretty far apart and the attraction is not always that strong. This is like saying the golf hole is not that deep. It happens, hence, that particles do escape from the nucleus in groups of four, as alpha decay. The theory of what nuclei will have what rate of alpha decay is then not very complex to compute.
The emission of gamma rays, which are photons, is also fairly straightforward, as it arises from energy differences either when the nucleus has gone to a higher energy level or when alpha decay leaves the daughter nucleus in an ‘excited’ state.
But the case of beta decay was not so simple. For one thing, it involved the transformation of what was considered to be an ‘elementary’ particle into another form. But a greater problem was that the energy of the emitted electron was not what it should have been, given the known energies of the neutron and the proton. Instead of being just the correct energy difference, the electron was seen to have energies ranging right from low values and up to the maximum possible. This did not make sense at all.
The German, Pauli first suggested that the reason may be that there was one more, mysterious, elusive particle being emitted, and which took away some of the energy. The Italian, Fermi, later developed a quantum mechanical theory where he actually showed that there should be a very low mass, neutral particle, which was christened the ‘neutrino’, emitted along with beta decay. The neutrino, in fact, was discovered years later, to confirm the Fermi theory and the neutrino became one more of a veritable menagerie of particles that were discovered over the years.
New particles had been discovered in the studies of cosmic rays and cosmic ray ‘showers’. Cosmic rays are mostly high-energy protons that stream in from outer space. These usually interact with the upper atmosphere and very rarely reach the surface of the earth. But in the course of interacting with the atmosphere, they give rise to ‘secondaries’, which do reach the earth’s surface.
|
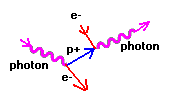
One frequent reaction is that a high-energy photon, a gamma ray photon, that is, would spontaneously split into an electron-positron pair. The positron was a particle exactly like the electron, except that its charge was positive. The positron was the ‘antiparticle’ of the electron, and while the pair could arise from a photon with sufficient energy, if the particles met, they would annihilate and give off a photon! As the total energy of the electron-positron pair, that is, the energy equivalent of their mass plus the energy due to their speed, had to come from the mother photon, pair production was seen only with high energy, gamma ray photons. |
|
|---|
The electron and positron, while coursing through the atmosphere, would meet up with other positrons and electrons, and on meeting, they would annihilate, in the form of new photons. The photons, in turn, could give rise to more e-p pairs and so on.
|
The photon, electron and positron were detected by the trails they left in ‘bubble chambers’. These were large cavities that contained a vapour ready to condense around energetic particles, like electrons, that may enter. As the electron and positron were charged particles, their motion would be affected by a magnetic field, just like a wire that carries an electric current is deflected by a magnetic field. By placing a magnetic field around the bubble chamber, the electron and positron would move in curved path, leaving curved trails and they could be identified. A similar thing happened with an electric field. These measurements helped find the mass of the particles as well. |
|---|
Apart from electrons and positrons, cosmic rays gave rise to a number of other particles, like mesons, or ‘intermediate’ mass particles, of nine different kinds and ‘hyperons’ of six kinds. Soon many other particles were discovered, in cosmic rays and when cosmic rays or the products of radioactivity were made to strike targets of various materials. The ‘subatomic zoo’ soon became well populated and schemes to classify ‘elementary particles’ took on the aspects of taxonomy!
The way Fermi ‘introduced’ a particle’ into the reckoning, to explain beta decay, was a very exact and refined form of quantum mechanics calculation. In calculations of the outcomes of things of daily experience, like the collision of billiard balls, the method used is to start with the speeds and directions of the system before the collision and then to see how the final state must be, given the need to conserve momentum, how the energy would be spent, depending on how elastic the balls were, and so on. In quantum mechanics, the ‘state’ of a system is described as the superimposition of ‘all possible states’, with some of these ‘possibilities’ being more likely to be seen, than others. It is this composite ‘state’ that evolves, under the influence of the energy in the system. In the case of large objects, the usual, familiar ‘states’ are by far the most likely. But at the subatomic scale, quantum effects’, of energy of a system increasing not smoothly but in ‘steps’, become important and even outcomes that involve ‘temporary’ violations of conservation of energy, are permitted. Quantum mechanics takes this into account and gives the correct results at the very small dimensions.
This method, to ‘quantise’ energy or momentum, led to spectacular success, particularly when applied to the electron going round a nucleus, in explaining the structure of the atom and the brightness of the colours emitted by atoms. A natural next step was to try and ‘quantise’ the electromagnetic field. The e.m. field is the distribution of electric and magnetic effects over a region of space. To ‘quantise’ the e.m. field was to consider that the energy in the field could not vary continuously, but only in steps. This kind of working things out led to the ‘photon’ or the particle of light, showing up as a natural property of the e.m. field. This dovetailed nicely with experiment, as the photon was already known, as a ‘quantum’ of e.m. energy, which could explain the way object radiated heat, or the phenomenon of light causing electric currents when it fell on certain metals.
A step from this quantum theory of the photon was to see whether all particles, like electrons, could be thought of as arising out of fields. Developing the properties of such fields, in lock-step with observation, soon grew into a powerful computational tool. Like a complex sound wave can be expressed as the sum of a series of simple waves that are harmonics of each other, the quantum fields could also be expanded as a series. It was found that the multipliers of the terms in this expansion were in fact ‘creators’ or ‘destroyers’ of particles, rapidly found confirmed in experiments!
The theory of beta decay had been developed as a form of the ‘weak’ interaction. Quantum theory has been able to explain three of the four main kinds of interactions seen in nature. The first, which quantum theory has not treated, is gravity, which is so weak that it is appreciable only between very large and heavy objects, like planets and stars. But the force acts even across very large distances. The next is electromagnetism, which attracts or repels charged objects and which keeps atoms and molecules together. Like gravity, this force has infinite range. The third and fourth kinds are very short range forces found inside atomic nuclei. The ‘strong’ force holds protons and neutrons together in the nucleus and is responsible for some kinds of radioactivity. But the last, the ‘weak’ force, is of the shortest range and is the one that leads to beta or other decays. It is called ‘weak’ because of its short range and because beta decay occurs more slowly than ‘alpha’ decay, which is caused by the ‘strong’ force.
The way the interactions are worked through is that in considering the energy of the system, a term is added to account for the interaction. An instance of an interaction is when a beam of positive particles is being scattered by the positive nucleus of a heavy atom. The energy of the system is due to the speed of the advancing particles and the repulsion of the beam by the nucleus. With this taken as the energy, the methods of quantum mechanics are able to work out the scattering that is actually seen. Similarly, to consider interactions involving the strong force, a term is added for the strong force. By comparing the results of different forms of the term, with results of experiments, the nature of the strong force can be worked out. But for consistent agreement with experiment, the term taken for the interaction needs to be of the correct form, in respect of its properties of direction, orientation and so on. The scientist, Pauli had shown that because of some conditions imposed by the theory of relativity, these interactions would have to be one out only five options – S - the scalar, P - the pseudoscalar, V - the vector, A - the axial vector or T - the tensor.
These options relate to how the interaction would be oriented, in relation to the position, motion or spin of the participants in the interaction. Fermi, in developing the theory of beta decay, had just considered the ‘vector’ form, because this had worked for the photon, and for some time, the theory was successful.
But then came further observations, of beta decay of particles that had a quality called ‘spin’. In a refined theory where this was included, the interaction needed to be A or T. Experiment seemed to support both V and T. Further experiment and theory showed that the interaction could not contain both S and V or both A and T. Further study also showed that either S or V and either A or T must also be present. But the experiments themselves were not always reliable and evidence was not clinching. In 1953, Petschek and Marshak (soon Sudarshan’s guide) concluded that it was T and P that gave the best fit and an ‘STP’ theory was current, till it was found that the P interaction was not necessary. The weak interaction stayed unknown territory.
It was about this time (1956) when two Chinese scientists Lee and Yang drew attentions to an assumption implicit in the work on weak interactions, that the interactions should conserve a property known as ‘parity’. This assumption, they said, may not be justified. Conservation of parity means that the laws of nature should not depend on whether we were left handed or right handed. Another way of putting it is that the world should work in exactly the same way even when seen through a mirror. This seems to be a natural condition and it does not look like there could be anything wrong with a theory that assumes that parity would be conserved

Conservation of parity, in fact, had been basic to the ‘universality’ of physical laws, an assumption that physics should be no different here than on a planet many light years away and with which we could not exchange the meaning of right and left.
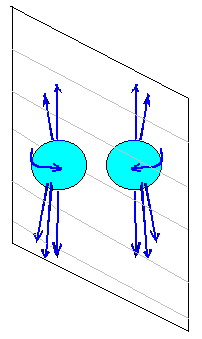
But the irony was that Yang and Lee did not find real evidence that beta decay conserved parity and they proposed an experiment to make sure. The experiment was the beta decay of the cobalt nucleus. Now, the cobalt nucleus has a property called spin, which marks it with a direction, like the North Pole, on the earth.
|
A bunch of such nuclei could be oriented with spins in the same direction. An experiment could now check the rate of beta decay. Only if the rate was the same in the direction of spin as in the opposite direction could parity be conserved On being seen through a mirror, directions of motion and position get reversed. In the case of a spinning nucleus, the direction of spin is reversed. But if rate of decay is different in different directions, then the mirror world is thus distinguishable from the ‘real’ world! |
|---|
Sudarshan was then a graduate student at Rochester, USA, working with Professor Marshak. His work was in the very field of ‘elementary’ particles, symmetries and computation of their masses and other properties. The suggestion that weak interactions may not conserve parity had just broken and experiment had shown that this indeed was the case. Sudarshan went hard to work and concluded first that there was no form of interaction consistent with the data. Some of the data hence had to be wrong. Second, with ‘spin’ accounted for, the form of interaction needed to include an axial vector component. The axial vector is like the momentum of a spinning top. The direction of motion of all points on the top, as well as their positions as measured from the axis of the top, get reversed on reflection. But the momentum of spin, which depends on both these entities, stays unchanged.
Marshak and Sudarshan, in the term for the interaction, put in a small component that was V and added a small component that was A. As the ‘A’ term would not change on reflection, there was a part of the interaction that would not change direction along with the others. Putting in this term would make sure that parity was violated. The actual terms had to be calculated so that the experimental numbers would come out right, without upsetting what was already working, which they did. And by 1957, they had the universal V-A interaction, for weak force, consistent with experiment, ready to present to the world.
What followed could be viewed, perhaps as not the best steps in bringing the fine work that had been done to the attention of the world. The work had been done by the time of the Rochester Conference, in 1957. But Sudarshan, as a graduate student, did not get to present the work and had to hear distinguished speakers puzzle over a problem that he had solved! A little later, Sudarshan presented to a gathering that included the celebrated Murrey Gell-Mann, how some of the experimental data was inconsistent and their own resolution of the puzzle through the V-A form of the interaction. Sudarshan and Marshak suggested that some of the experiments be redone, which led to some of the experiments being proved faulty and the V-A form as correct theory. In September 1957, Marshak presented a paper at a conference in Padua-Venice and they considered their finding as good as published. This, in retrospect, was an error. Sudarshan did try to ask Marshak to get the work formally published, but he was then a student, and Marshak appears to have been preoccupied.
A little after Marshak and Sudarshan’s work, Richard Feynman and Gell-Mann who had worked on weak interactions from a field theory viewpoint also proposed a V-A form for beta decay, in a paper in Physical Review. A paper in Physical Review was certainly a more public presentation and it is not surprising that for some years, it was Feynman and Gell-Mann who got the limelight for the V-A theory of the weak force.
In the last forty years, the theory of elementary particles has got greatly refined, with the proton itself considered to be made of three of a set of particles called ‘quarks’, which give rise to the strong force by exchange of ‘gluons’, and the weak force is seen to arise from exchange of very heavy, ‘W’ particles. In perspective, Sudarshan’s early work leading to the V-A interaction being responsible for the weak force has been seen as the start of much of the development and now, at least, his place in the story is unquestioned.
Sudarshan got his Ph.D. shortly after and he moved on to work at Harvard, Rochester, Berne, Syracuse and from 1969, at University of Texas at Austin. For eleven years, he was senor professor at the Indian Institute of Science, Bangalore and for six years, Director of the Institute for Mathematical Sciences, Chennai. He continued to work in different fields and wrote a book on particle physics, along with Professor Marshak.
He is married to Professor Bhamathi, also a physicist, and has three children.
------------------------------------------------------------------------------------------