The Day of Pi (appeared in March 2015

There is higher math hidden behind the simple ratio of the circle to its radius, says , says S.Ananthanarayanan.

A special day to celebrate the number, pi, denoted by the symbol,, and known to most as 22/7 or the value 3.14, in decimals, was observed on the 14th of March, last week. This was in the US and such places, where the date is written month-first, as 3/14, which reads out the usual value of pi. And 14th March 2015 is even more special, because the date reads as 3/14/15, which gives the value of pi to four decimal places and happens only once in a century. Even more memorable was the date, 14th March 1592, four centuries ago, because 3.141592 is the value of pi to six decimal places! But even in our own century, “mass elation will peak at 9.26:53 am when the date and time will describe pi to 10 digits, said the newspaper, the guardian. “This only happens once in 100 years, so it is likely your only chance to celebrate,” says the ‘learning’ part of the website of the Bangkok Post.

But apart from being a mathematical value that fits into the calendar in this way, the number pi has features that make it quite a star, even otherwise, among mathematical things. The purpose, of naming a day after the number pi, and celebrating the day, by eating pies, for instance, one hopes, is to use fun and food to draw attention to different qualities of this important number.

The first property of the number, of course, is that it is the ratio of the diameter of a circle to the circumference [c=2r]. The fact that it is also the ratio of the square of the radius to the area of the circle[area =r²] would suggest how this ratio arises. But at first, it was worked out simply as the constant ratio that masons and carpenters had noticed in the course of their work, and this value is approximate, but practical. It is perhaps Archimedes who first used a mathematical method to work out the ratio without recourse to actual measurement of circles. This way of working things out is called the analytical method.

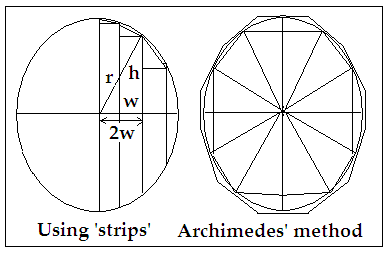
One direct way of doing this is to divide the circle into strips, like in the picture. Now, if each strip is taken as having some width, then the distance of the strip from the centre is known and hence, using principles of geometry, its height is also known. With the help of the height and the width, we can work out the area of each strip. And then, adding together the area of all the strips leads us somewhat near the area of the circle. Now, if we make the strips narrower and narrower, we can work out the area of the circle more accurately, in terms of the radius. We can similarly, workout the very nearly the circumference of the circle, by adding up all the slanting ends of the strips, as the strips get narrower and narrower. Archimedes actually did something like this, only he worked out the area, or the sides, of a polygon, which is a many sided shape, fitted inside and outside the circle, and then increasing the number of sides, to make the shape more nearly like the circle.

In later centuries, with the advance in mathematics, the principles of these methods have been refined and we have exact formulas. The Liebniz (1646-1716) formula, for instance, gives the value of pi as : p/4 = 1 - 1/3 + 1/5 - 1/7 + 1/9 – 1/11+ 1/13 -…….. to infinite terms. We can notice a feature of this series, that every odd, and positive, term is slightly greater than the adjacent, negative term. The value of the series hence gradually increases, to approach an exact value of pi, the addition by the terms further down in the series being smaller and smaller. But the trouble with this formula is that the approach to the correct value is slow, and it takes many, many terms before the value is good enough. Better formulas have been developed, by different ways of working out the areas in the elementary strips or polygons, and these are now used for the most accurate computation of the value of pi.

One method was even an ‘experimental method’ – of dropping a needle on to a grid of parallel lines and counting the number of times the pin fell over a line. It has been worked out that if the lines are drawn one unit apart and the length of the pin, k, is less than this unit, then the probability that the pin will cross a line is 2k/p. Throwing the pin, which can be automated, a huge number of times can thus generate an accurate value for p.

Is the value important?

The value, beyond a few decimal places, is of no practical value, except in large surveys or astronomy, where more decimal places are needed. But there is great academic interest in the fact that the exact value of p can never, ever, be evaluated. This is because the correct value is an infinite series of non-repeating digits after the decimal point, never evaluating to an exact value. Such a quality of a number amounts to saying that it can never be correctly expressed as a ration of two integers, which are not multiples of each other, like 7/3, or 13/5 or even 22/7. All fractions like this, where one whole number divides another, can be evaluated as an exact decimal number, or as a recurring decimal, where the division keeps giving the same remainder or the same series of remainders. Examples of recurring decimals are 1/3= 0.3333333… or 31/99= 0.31313131… .

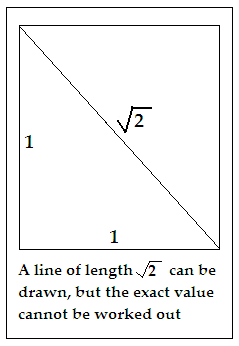
Numbers like this, which cannot be expressed as a ratio, are called irrational numbers. There are more examples, a ready one being the square root of the number 2. This example is easily proved, as in case this square root could be expressed as a ratio, then the squares of the two integers in the ratio would divide into the number 2. And there is no such pair of squares.

Random progression

An important application of this kind of number, whose decimal expansion is infinite, is that the progression of digits in the decimal form is essentially random. This must be so, as else, there would be a endless repetition of patterns. Irrational numbers are thus a source of randomness, and a computer generated value, running into millions of digits, could be used as a code that it would be very difficult to break, unless the eavesdropper knew at which stage of the progression the code maker had started.

These properties of pi and other irrational numbers are the same even if we change the system of counting from the decimal, or on the base of 10, to another, like the binary, based on 2, or the Octal, based on 8, or the hexadecimal, based on 16, which computers use. The properties, in fact represent basic features of circles, lines and angles, and even geometries in more than three dimensions and the study of the number pi is of great and fundamental importance.

Value of Pi A mnemonic for the value of pi to 11 digits goes like this: “Now I know a rhyme excelling, of mystic words and magic spelling.” The number of letters in the words of this mnemonic provide the integers in the value of pi as: 3.14159265358 Computers, of course, can do much better: Pi = 3.1415926535 8979323846 2643383279 5028841971 6939937510 5820974944 5923078164 0628620899 8628034825 3421170679 [Pi to 100 decimal places] OR 3.1415926535 8979323846 2643383279 5028841971 6939937510 5820974944 5923078164 0628620899 8628034825 3421170679 8214808651 3282306647 0938446095 5058223172 5359408128 4811174502 8410270193 8521105559 6446229489 5493038196 [pi to 200 decimal places]

------------------------------------------------------------------------------------------

Do respond to : response@simplescience.in