Limiting spread of an epidemic needs strategic action, says S.Ananthanarayanan.

Administrations around the world have devised ways to control the spread of COVID-19. They have uniformly found isolation, and cutting the transmission chain, to be the only answer.

The growth in the numbers has been described as exponential. When we say a quantity is increasing ‘exponentially’, we mean that the quantity is increasing like a bank deposit with ‘compound interest’ – the increase in the balance becomes greater every year. And this is because the increase is based not on what was deposited, but on the balance at the start of each year.

In the case of COVID-19, we are told that each case, while it lasts, would infect some 2.3 other cases, on the average. One case would hence be 2.3 cases at the end of two weeks. Two weeks later, the number would grow to 2.3 x 2.3 = 5.29. And two weeks later, we would have 5.29 x 2.3 = 12. This means one case that we started with has grown to 12 in six weeks. Two weeks later, the number is 28 and in another two weeks, it swells to 148. In the same period that a single case grew to 148, these 148 would grow to 21,915.

We can see that, as long as each case infects even just a little more than one other, the numbers can rise very fast. And with even low levels of consequences, like death or need for onerous medical care, large numbers would not be acceptable.

Exponential increase takes place when things multiply by reproduction, like microbes in laboratory culture, or weeds in a pond. And so long as the culture tray, or the pond, has space for expansion, the growth stays exponential. However, when the space available begins to shrink, the population uses up resources and the rate of increase reduces. In the case of COVID-19, persons who are infected would not reduce the potency of the virus. The rate of spread, however, would still fall, when the infection has progressed, because some of the 2.3 persons who could be infected may be already infected, or have just recovered and are able to resist infection.

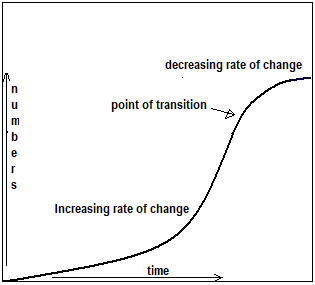
Exponential growth can be shown graphically as in the picture, where the curve grows increasingly steep, to show the increasing rate of change as the numbers grow higher. But towards the end, the limiting features kick in and the rate of increase falls, and the curve ‘flattens’

A few years ago, Colin Adams, a professor of mathematics, examined the same question in his book, Zombies and Calculus. In the place of COVID-19, there was a virus that changed people into zombies – or humans, with all the organs of humans, but devoid of rational thinking, and driven by a compulsion to bite and infect other humans. These other humans then turn into zombies and go out to infect more humans.

p>

The action is in a small liberal arts college in Massachusetts, where zombies are at large, and a professor of mathematics guides the community to survive and overcome the attack. While the book brings in many features of mathematics to help in the struggle, a significant point it makes is that as more humans are infected and the number of zombies increases, there are less targets the zombies can hit and the rate of spread must fall. The book resolves the problem with the zombies being limited to one area of the earth, while normal humans occupy another area, effectively with the zombie population being excluded from society.

Heading

Matter with border line 1
Line 2.

This is paragraph 1, yes it is...

The image will appear along the...isn't it?

This is the third paragraph that appears...

------------------------------------------------------------------------------------------ Do respond to : response@simplescience.in -------------------------------------------